W. D. Bauer's
calculations of his parametric overunity rotator -
simply refuted
By G. W. Bruhn,
Darmstadt University of Technology
Abstract: After several updates in the past, W.D. Bauer released
a new update of his note on the overunity properties of his parametric
overunity rotator [1] recently. Though W. D. Bauer knows about the
following very simple arguments against his new calculations by private
communication, he insists upon being right, against all evidence. The
following short note is an excerpt from a longer article [2], where the author
showed that there are no OU effects at all in W.D. Bauer’s devices.
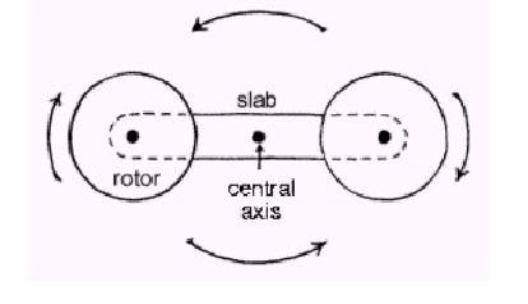
W.D. Bauer’s
rotator consists of a rotating slab, the angular position of which in the plane
is denoted by ![]() , and of a pair of rotors mounted symmetrically on the
slab the angular position of which in the plane is denoted by
, and of a pair of rotors mounted symmetrically on the
slab the angular position of which in the plane is denoted by ![]() . Separate
driving motors on the central axis control the speed of both slab and rotors by
means of belts. Let
. Separate
driving motors on the central axis control the speed of both slab and rotors by
means of belts. Let ![]() and
and ![]() denote the inertia moments of slab and the
rotor pair, respectively. For further information the reader is
referred to [1].
denote the inertia moments of slab and the
rotor pair, respectively. For further information the reader is
referred to [1].
During a working
cycle the rotator device travels through three phases: During
phase 1 both the slab and the
rotors are accelerated from rest to angular velocities ![]() and
and ![]() , respectively.
During phase 2 the angular velocities
are equalized by different methods. During phase 3 the whole device rotating with
common angular velocity
, respectively.
During phase 2 the angular velocities
are equalized by different methods. During phase 3 the whole device rotating with
common angular velocity ![]() is brought back to rest by detracting its
kinetic energy.
is brought back to rest by detracting its
kinetic energy.
To show W. D. Bauer’s result to be erroneous we
consider phase 2.
1.
The
driven rotors device
Let us first discuss the special case h =
1: At the beginning of phase2 the slab is decoupled from its drive motor such
that it can rotate freely with its angular velocity ![]() due to its inertia.
The rotors are rotating with the angular velocity
due to its inertia.
The rotors are rotating with the angular velocity ![]() , which has to be reduced by the rotor’s drive motor to the
final smaller value
, which has to be reduced by the rotor’s drive motor to the
final smaller value ![]() at the end of phase
2. Evidently this can be done by
detracting the difference of the rotational energies via the rotor’s drive
motor, with other words, the negative amount of energy
at the end of phase
2. Evidently this can be done by
detracting the difference of the rotational energies via the rotor’s drive
motor, with other words, the negative amount of energy
![]()
has to be imported to the system, i.e. we
obtain an energy gain. Instead of this, W.D. Bauer calculates the amount of
energy (see (14) in [1])
![]()
by a
complicated and dubious method. His result ![]() means, in
contrast to all evidence, that a positive amount of energy has to be imported
to the system via the rotors, in spite of the fact, that there is already
a surplus of energy contained in the rotors.
means, in
contrast to all evidence, that a positive amount of energy has to be imported
to the system via the rotors, in spite of the fact, that there is already
a surplus of energy contained in the rotors.
The same consideration applies to all values of
![]() and all
and all ![]() . Thus we
conclude that W.D. Bauer’s formula (14) for the energy
required for phase 2 (and hence his total energy balance (19)) is
erroneous and cannot be true, while our result above is confirmed in
[2].
. Thus we
conclude that W.D. Bauer’s formula (14) for the energy
required for phase 2 (and hence his total energy balance (19)) is
erroneous and cannot be true, while our result above is confirmed in
[2].
2. A contradiction in W.D. Bauer’s treatment of
the braked rotors device
W.D. Bauer
discusses two other possibilities of equating the angular velocities of the
rotors and the slab. The rotors are now braked against the slab:
A.
By braking after having decoupled
the slab from its driving motor.
B. By braking while
the slab rotation is kept at ![]() constantly by its driving motor.
constantly by its driving motor.
Case A: For the braking
process of the rotors against the free slab W.D. Bauer obtains an energy
loss during phase 2 of the size
![]()
(cf. (32) in [1]). We have checked this result and agree with
it.
Case B: The braking is
executed against the slab that is kept at constant ![]() by its driving motor. We can approximate case
B by case A, if we assume the slab to have a very large momentum of inertia,
i.e. if
by its driving motor. We can approximate case
B by case A, if we assume the slab to have a very large momentum of inertia,
i.e. if ![]() . Then the
braking process will, due to the very large value of
. Then the
braking process will, due to the very large value of ![]() , not
considerably influence the slab, i.e. the slab will approximately keep
its angular velocity
, not
considerably influence the slab, i.e. the slab will approximately keep
its angular velocity ![]() , with other
words, the slab condition for case B is approximately fulfilled. Therefore we
can expect to obtain the energy balance
, with other
words, the slab condition for case B is approximately fulfilled. Therefore we
can expect to obtain the energy balance ![]() of phase 2 by neglecting
of phase 2 by neglecting ![]() in the denominator of the formula of
in the denominator of the formula of ![]() above. This yields
above. This yields
![]() .
.
as the energy balance of case B, which is an energy
loss again as in case A. But W.D. Bauer’s result (43) in [1] is ![]() , which is negative, i.e. a gain of energy, for
, which is negative, i.e. a gain of energy, for
![]() , a result, that
is very amazing in view of the preceding case A. Therefore we cannot expect W.D.
Bauer’s result (43) to be true, while our result above is confirmed
in [2].
, a result, that
is very amazing in view of the preceding case A. Therefore we cannot expect W.D.
Bauer’s result (43) to be true, while our result above is confirmed
in [2].
References
[1]
W.D. Bauer: The parametric overunity rotator – the Wuerth power booster, (with
last corrections on 3.10.00) at http://www.overunity.de/rotator/rotator2.htm
[2] G.W. Bruhn: W. D. Bauer's parametric overunity rotators - devoid
of all overunity, available for the time being via W.D. Bauer’s References in
[1]
The author: bruhn@mathematik.tu-darmstadt.de